Intervals are special subsets of the set of real numbers.

An interval is represented by a line segment on the number line.

An open interval contains all points between two endpoints; the endpoints are not included in the open interval.

Open interval  (a, b)  is the set of points  x
such that  a < x < b.

An open interval of the form (a – δ, a + δ)  is called a δ–vicinity of the point  a. It is shown in the figure below.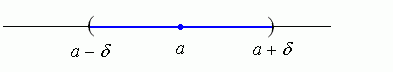

A half-open interval contains all points between the endpoints and one of the endpoints.

Half-open interval  [a, b)  is the set of points  x
such that  a ≤ x < b.

Half-open interval  (a, b]  is the set of points  x
such that  a < x ≤ b.

An interval is called closed if both endpoints are included in the interval.

Closed interval  [a, b]  is the set of points  x
such that  a ≤ x ≤ b.

An infinite interval is the set of all real numbers. It has no endpoints and so infinity symbols are enclosed by round brackets, .

A half-infinite interval is a set of real numbers represented by a part of the number line bounded from one side and unbounded from the other, in the direction of positive or negative infinity.

A half-infinite interval has only one endpoint, and it may be open or closed at the endpoint.

Index Real Numbers Complex Numbers Exponentiation Algebraic Transformations Algebraic Equations and Ineualities Functions Discrete Algebra Basic Formulas Graphics of Basic Functions