About Algebra
Addition and Subtraction
Multiplication
and Division
Criterions for Divisibility
 Types of Numbers
Geometric Interpretation of Real Numbers
Irrational Number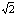 
Properties of Real Numbers
Fractions
Proportions
Property of Equal Proportions
Absolute Values
Graphical Illustrations
Sets
Subsets
Operations with Sets
Intervals
|
|
|
An even number is divisible by 2.
A number is divisible by 3 if it is formed of digits, the sum of which is a number divisible by 3.
A number is divisible by 4 if its two last digits form a number, which
is divisible by 4.
A number is divisible by 5 if its the last digit is zero or 5.
A number is divisible by 6 if it is divisible by 2 and by 3 at the same time.
A multidigit integer is divisible by 8 if its three last digits form
a number, which is divisible by 8.
A number is divisible by 9 if it is formed of digits, the sum of which is a number divisible by 9.
A number is divisible by 10 if its the last digit is zero.
A multidigit integer is divisible by 25 if its two last digits form
a number, which is divisible by 25.
A number is divisible by 100 if its two last digits are zeros.
|
