| Property 1 |
|
The product of an infinitesimal sequence and a
bounded sequence is an infinitesimal sequence.
|
Explanation: The
absolute values of the terms of a bounded sequence 
are restricted by a finite positive
number M, that is,
for each natural n.
If 
is an infinitesimal variable then
.
| Property 2 |
 |
|
The sum of two infinitesimal sequences is an
infinitesimal sequence
|
Explanation:
If 
and 
are infinitesimal sequences then
.
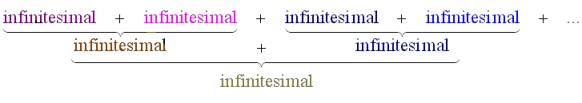
| Property 2 Corollary |
|
| The sum of any finite number of infinitesimal sequences is an infinitesimal sequence |
Explanation: An idea of a proof is shown in the drawing below, namely, the sum of two infinitesimals is an infinitesimal, the sum of which and a third infinitesimal is also infinitesimal, etc.
| Property 3 |
|
|
The sequence inverse of an infinitesimal sequence is infinite large; |
Explanation: To verify these propositions, divide number one by 1000, 1000000, 1000000000, and so on. Then divide number one by 0.001, 0.000001, 0.000000001, and so on.
