The sequence

is infinitesimal, since

as .

Formal Proof: We need to show that for any there exists a number N such that the inequality implies

.

Let integer N be greater than or equal to .

Then inequality

implies ,

which means

for any arbitrary small .

Hence, the desired statement.

If we set then for all .

Therefore, all terms of the sequence are in the given delta vicinity of zero with the exception of the first hundred of the terms.

If then for all .

Therefore, all terms of the sequence are in the delta vicinity of zero with the exception of the first thousand of the terms.

It does not matter how many terms are not in the delta vicinity. It is only important that a finite number of terms is outside of any neighborhood of zero.

Example 2

The variable is infinitesimal, since

as .

.

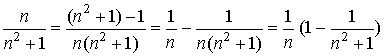Next, if we set

,

then for any inequality implies , which means .

However,

.

Therefore,

for any arbitrary small and positive .

Hence, the desired result.

Example 3

The variable is infinitesimal, because

as .

.

Hence, we can set

,

and so inequality implies

for any arbitrary small .

Example 4

The variable is infinitesimal, because

as .

.

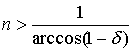
Let integer N be greater than or equal to .

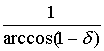Then inequality implies

for any arbitrary small .